NEW SCIENCES FOR A NEW ERA:
Mathematical, Physical and Chemical Discoveries of
Ruggero Maria Santilli
I. Gandzha and J Kadeisvili
PRINTED VERSION
I. Gandzha and J Kadeisvili, New Sciences for a New Era:
Mathematical, Physical and Chemical Discoveries of
Ruggero Maria Santilli,
Sankata Printing Press, University of Kathmandy, Nepal (2011),
Free pdf download
http://www.santilli-foundation.org/docs/RMS.pdf
CHAPTER 2:
DISCOVERIES IN MATHEMATICS
2.1. FOREWORD
2.2. DISCOVERY OF NEW NUMBERS
2.2A. Discovery of isonumbers (1983)
2.2B. Discovery of genonumbers (1993)
2.2C. Discovery of hypernumbers (1994)
2.2D. Discovery of isodual numbers (1993)
2.3. DISCOVERY OF ISO-, GENO-, HYPER-DIFFERENTIAL CALCULI, FUNCTIONAL ANALYSIS AND THEIR ISODUALS (1996)
2.4. DISCOVERY OF ISO-, GENO-, HYPER-, SPACES AND THEIR ISODUALS (1983).
2.5. DISCOVERY OF ISO-, GENO-, HYPER-SYMPLECTIC GEOMETRIES AND THEIR ISODUALS (1996)
2.6. ISOTOPIC UNIFICATION OF MINKOWSKIAN, RIEMANNIAN AND FINSLERIAN GEOMETRIES (1998)
2.7. LIE-ISOTOPIC COVERING OF LIE'S THEORY AND ITS ISODUAL (1978)
2.8. LIE-ADMISSIBLE COVERING OF THE LIE-ISOTOPIC THEORY AND ITS ISODUAL (1967)
2.9. INTEGRABILITY CONDITIONS FOR THE EXISTENCE OF A LAGRANGIAN
2.9A. Integrability conditions in Newtonian mechanics (1978).
2.9B. Integrability conditions in field theory (1975)
CHAPTER 2:
SANTILLI'S DISCOVERIES IN MATHEMATICS
2.1. FOREWORD
Santilli has repeatedly stated that: The origin of protracted controversies or unsolved problems in physics, chemistry, biology, and other sciences, is generally due to the use of mathematics basically insufficient for the quantitative treatment of the problem at hand, with consequential need to develop new appropriate mathematics.
Most of the insufficiencies of the 20th century theories identified in the preceding chapter see their origin precisely in the lack of adequate mathematics, such as: the reconstruction of an algebra in the brackets of the time evolution of the analytic equations with external terms, Eq. (1.3), clearly requires the development of a suitable new algebra other than Lie algebra; the classical and operator treatment of nonlinear, nonlocal and non-Hamiltonian interactions for extended particles at short mutual distances (figure 1.3) clearly requires a mathematics broader than that effective for conventional Hamiltonian and quantum theories; the insufficiencies of curvature to represent gravitation, combined with the inabilities by general relativity to reach a grand unification and a quantum version of gravity dating back to Einstein's times without solution, are a clear manifestation of the need for a more appropriate geometry for gravitational events; and the same occurs for other problems whose solution is impossible with the mathematics of the 20th century.
Santilli has also stated repeatedly in his writings that: There cannot be a really new theory without a really new mathematics, and there cannot be a really new mathematics without new numbers.
Hence, as a theoretical physicist, he devoted the majority of his time to the search of new numbers, and then to the construction of new mathematics based on them. Discoveries in physics, chemistry, biology, astrophysics and engineering required the minority of his time.
To understand the mathematical discoveries outlined below, one should keep in mind the main problem investigated by Santilli. Recall from Section 1.1 the legacy of Lagrange and Hamilton according to which the representation of nature requires the knowledge of two quantities, a Lagrangian or a Hamiltonian and the external force F(t, r, p, ...). Recall also from Eq. (1.3) that the presence of the external forces causes the loss of all algebras in the brackets of the time evolution of physical quantities, thus preventing the construction of physically meaningful covering theories.
Hence, Santilli set his research goal to identify an identical reformulation of Hamilton's equation (1.2) depending on a second quantity, besides the Hamiltonian, capable of restoring an algebra in the brackets of the time evolution and that algebra had to be a covering of the Lie algebra.
After extensive research and the systematic investigations of all possible alternatives, Santilli finally assumed the representation of Lagrange's and Hamilton's external forces via a generalization of the basic unit into a form explicitly dependent on local variables generally used in physics, hereon denoted I*(t, r, p, E, ...). All other alternatives failed because of their lack of invariance over time, that is, the inability to predict the same numerical values under the same conditions at different times, thus being physically inconsistent.
By comparison, the unit is the most fundamental invariant of all theories, thus being the best solution for the preservation of the same time invariance as that of the truncated analytic equations. However, the generalization of the basic unit requires a corresponding, progressive, and systematic generalization of the totality of the mathematics of the 20th century, and this explains the dimension as well as novelty of Santilli's mathematical discoveries.
In this chapter we outline the rudiments of Santilli mathematics, at times also called hadronic mathematics to indicate the mathematics underlying hadronic mechanics, namely, we shall outline the formulation of numerical fields, vector and metric spaces, geometries, algebras and groups, etc., when characterized by a basic unit I*(t, r, p, E, ...) that, besides being nowhere singular, has otherwise an unrestricted functional dependence on all needed local variables. The application of Santilli mathematics for the resolution of Lagrange's and Hamilton's legacy is outlined in Chapter 3.
Mathematicians should be aware that all mathematical discoveries outlined in this chapter originated from specific physical needs following clear insufficiencies of the pre-existing mathematical and physical methods. Mathematicians should also keep in mind that Santilli has been a member of the Department of Mathematics of Harvard University from 1978 to 1983 under DOE financial support, thus having all qualifications for mathematical discoveries even while being a theoretical physicist. Nevertheless, mathematicians should keep in mind that, except a number of papers written in pure mathematics language for mathematicians, numerous mathematical discoveries were presented by Santilli in papers intended for physicists and published in physics journals, the understanding being that their re-elaboration in the language of pure mathematics is elementary.
n this mathematical chapter, the conventional associative multiplication "ab" of two generic quantities a, b (such as numbers, matrices, operators, etc.) will be denoted with the symbol "a x b" in order to differentiate it from various new multiplications discovered by Santilli that are still associative, yet more general than the trivial multiplications ab.
A main criticism ventured in academia interested in preserving old mathematics (but not in the industry using new mathematics) is that {\it Santilli's new mathematics is trivial because it boils down to putting a "hat" on symbols without major changes of pre-exciting axioms.} As we shall; see, the statement is technically correct because Santilli's various different mathematics can indeed be presented by putting "hats" or other indices in pre-existing mathematical symbols.
However, on mathematical grounds the statement is dishonest when proffered by expert mathematicians because the implications are far from being trivial. For example:
A) The placement of Santilli's "hat" (meaning isotopy) on the symbols of Lie's theory allows the extension of the applications from linear systems to all possible (well behaved) nonlinear systems, a discovery far from being trivial;
B) The placement of Santilli's "hat" in the symbols of the symplectic geometry renders the same universal for the characterization of all possible (well behaved) non-Hamiltonian system directly in the frame of the experimenter and without any sue of the transformation theory, a result with historical mathematical significance (since the symplectic geometry is rendered directly universal) and industrial implications (since for the first time the optimal control theory is applicable to the real non-Hamiltonian systems of our real environment);
C) The placement of Santilli's "hat" in the symbols of special relativity extend its its applicability from dynamics in vacuum to dynamics within physical media with implications such as the elimination of "dark matter" and "dark energy", the development of basically new, environmentally clean and cost competitive new fuels and energies (Chapter 9), a discovery of clear historical proportion and implication rather than of trivial character.
In reality, the greatness of Santilli's mathematical discoveries, ignored by equivocal mathematicians under the illusion of personal gains, has been precisely that of achieving a formulation of his various new mathematics that coincides at the abstract realization-free level with conventional mathematics, thus essentially discovering {\it new realizations} of pre-existing abstract mathematical axioms.
2.2. DISCOVERY OF NEW NUMBERS
2.2A. Discovery of isonumbers (1983)
Numbers are at the foundation of all quantitative sciences since, by definition, the latter require mathematical elaborations predicting numbers that can be verified with experiments. For various topological and other technical reasons, experimental measurements requires the adopted "ordinary numbers" (hereon referred to those with characteristic zero and denoted with the letter n) to verify the axioms of a numerical field F(n, x, I) with associative multiplication n x m, (left and right) multiplicative unit I, I x n = n x I = n, addition n + m = p ε F and additive unit 0, 0+n = n+0 = n, ∀ n, m ε F.
The achievement of the modern number theory required
contributions from the best scientific minds in history, including Gauss, Legendre, Jacobi, Cauchy, Lebesgue, Diriclet, Hamilton, Cayley, and many others.
A major historical effort was dedicated to the classification of all possible numbers, that is, all possible sets verifying the axioms of a numerical field. By the middle of the 20th century, it was universally believed in mathematics that the classification of all ordinary numbers (again, those with characteristic zero) had been achieved with the results that all possible ordinary numbers are given by real numbers, complex numbers and quaternionic numbers. Octonions do not qualify as numbers because they violate the associativity of the multiplication (m x n ) x p = m x ( n x p).
As part of his Ph. D. in theoretical physics in the late 1960s at the University of Torino, Italy, Santilli set up his research goal of achieving a generalization-covering of quantum mechanics for which, to avoid the illusion of a real generalization, he needed numbers more general than those used in quantum mechanics, such as the real and complex numbers.
The difficulty of Santilli's task was that, on one side, very authoritative mathematicians claimed emphatically that all ordinary numbers verifying the axioms of a numerical field had been classified while, on the other side, Santilli needed new numbers verifying indeed said axioms to avoid physical inconsistencies identified later on.
With great scientific audacity, and based on the conviction that mathematics will never admit final formulations, Santilli ignored all authoritative claims and set himself up to review the foundations of number theory. His position at the Department of Mathematics of Harvard University proved to be instrumental, not because of any help by departmental colleagues, but because their skepticisms reinforced his determination.
In this way, Santilli first discovered that the axioms of a numerical field do not require that the multiplicative unit be necessarily the number I = +1 dating back to pre-biblical times but used in pure mathematics up to the 20th century, since the left and right multiplicative unit can be an arbitrary positive-definite quantity I* = 1/T > 0 generally outside the original set F(n, x, I), provided that the multiplication is suitably re-defined in the form n x* m = n x T x m, under which I* remains indeed the correct left and right unit, I* x* n = n x* I* = n for all elements of the set.
Santilli then proved that, under the above assumptions for the multiplication and its unit while keeping the conventional addition and its unit, all axioms of a field were verified even when the new unit I* is not an element of the original field F, in which case the new numbers are written n* = n x I*. We reach in this way new numbers and fields for which Santilli suggested the name of isonumbers and isofields. from the Greek meaning of preserving the original axioms. They are known today as Santilli isoreal, isocomplex and isoquaternionic numbers, or generically isonumbers, the new unit I* = 1/T > 0 is called Santilli isounit, its inverse T is called the isotopic element, and the new multiplication a x* b between two generic quantities a, b is called isomultiplication. The new sets F* are called Santilli isofields and are generally written in the form
In short, Santilli discovered a new realization of the conventional axioms of a field permitting new physical, chemical and other applications identified in subsequent chapters. When the new unit I* is outside the original set F, F*(n*,x*, I*) is called an isofield of the first kind, and the numbers n* are called isonumbers of the first kind. When I* is an element of the original field F, that is, an ordinary number, F*(n*,x*, I*) is called an isofield of the second kind, in which case the isonumbers of the second kind are often assumed to be the original numbers n without the multiplication by I*.
Santilli's isofields of the second kind are primarily used in mathematics, particularly to show the insufficiency of contemporary number theory, and its various notions such as that of prime (see the example below). Santilli's isofields of the first kind are of primary use for the new non-Hamiltonian classical and operator mechanics and their applications. Therefore, unless otherwise stated, in this book we shall always use Santilli's isofields of the first kind and simply refer them as "isofields."
Even though isofields are isomorphic to conventional fields, as indicated by their very name and Eq. (2.2), their differences are by far nontrivial, and their scientific implications beyond our imagination at this time. For instance, fo the general isofields (of the first kind) we have expressions of the type
These results signaled one of the biggest mathematical discoveries of the 20th century because it gave rise to momentous advances in physics, chemistry, biology and other quantitative sciences reviewed in the subsequent chapters.
Quite symptomatically, Santilli published for the first time his isonumbers in his two historical papers of 1985 on the isotopies of Lie's theory, particularly for the structural lifting of the fundamental symmetry of physics, the rotational symmetry that, in turn, is the basis for his lifting of Galilei's and Einstein's relativities:
Lie-isotopic liftings of Lie symmetries, I: General considerations
R. M. Santilli,
Hadronic J. Vol. 8, 25-35 (1985)
Lie-isotopic liftings of Lie symmetries, II: Lifting of rotations
R. M. Santilli,
Hadronic J. Vol. 8, 36-51 (1985)
A mathematically rigorous presentation of isonumbers and isofields was then given in the 1993 paper
Isonumbers and genonumbers of dimension 1, 2, 4, 8, their isoduals
and "hidden numbers" of dimension 3, 5, 6, 7.
R. M. Santilli, Algebras, Groups and Geometries Vol. 10, 273-322 (1993).
A comprehensive study was then presented in the monograph
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1993).
Numerous independent papers and books have been written on Santilli isonumbers and isofields (see the General Bibliography). We here merely quote the monograph written in 2001 by the Chinese mathematician Chun-Xuan Jiang that remains a significant general study in the field to this day
"Foundations of Santilli Isonumber Theory"
Chun-Xuan Jiang, International Academic Press (2001).
A readable presentation of Santillis isonumbers in Italian is given by the paper
I nuovi numeri santilliani,
Parte I: I nuovi numeri isotopici e loro applicazioni
Michele Sacerdoti, to appear.
2.2B. Discovery of genonumbers (1993)
Despite the dimension and implications of the preceding discovery, Santilli remained dissatisfied because his main objective was to reach a structural generalization of quantum mechanics suitable for the representation of energy releasing processes, such as nuclear fusions, that are irreversible over time (that is, the time reversal images violate causality laws). Isonumbers could not allow such a generalization because they have no "time arrow."
Hence, Santilli went back to work and re-examined the foundations of his own isotopic number theory. He discovered in this way that, in addition not to require the value I = +1 for the multiplicative unit, the axioms of a field do not require that the unit for the multiplication to the right be equal to the unit for then multiplication to the left, provided that all multiplications are correspondently ordered to the right and to the left, respectively.
This discovery gave rise to a broader class of new numbers (again with characteristic zero, the sole known to have physical applications at this writing), also verifying the axioms of a field, called by Santilli genonumbers in the Greek meaning that they induce a new structure. The new numbers are known today as Santilli's genoreal, genocomplex and genoquaternionic numbers to the right and to the left or, generically, as genonumbers..
By using the symbols If and n xf m for the genounit and genomultiplication to the right (physically interpreted as forward in time) and the symbols bI and n bx m for the genounit and genomultiplication to the left, (physically interpreted as backward in time) we can write the genofields in the form
where c is a conjugation depending on the desired application (such as Hermitean conjugation. complex conjugation, inverse, transpose, etc.) needed for the interconnection between the right and left genofields.
Again, genofields are isomorphic to conventional fields by conception and construction. Nevertheless, the implications are by far nontrivial. For instance, by using the inverse for the conjugation c, a generic realization of Ff(n, xf, If) and bF(b, bn, bI) is given by
namely, not only the product of 2 times 3 does not yield the usual number 6, but the product to the right is different than that to the left, all in a way fully compatible with the axioms of a numerical field.
This discovery carries scientific implications greater than those originating from isonumbers, because genonumbers have permitted the construction of mathematically rigorous methods for the invariant treatment of irreversibility, including the study of new energies, that are not treatable with the mathematics of the 20th century, because the latter has no "time arrow". In fact, Santilli genotheories represent irreversibility via the most basic mathematical quantity, the unit, with the physical interpretation that genounits and genomultiplication to the right (left) represent motion forward (backward) in time.
Santilli presented the discovery of genonumbers in his historical mathematical paper of 1993
Isonumbers and genonumbers of dimension 1, 2, 4, 8, their isoduals
and "hidden numbers" of dimension 3, 5, 6, 7.
R. M. Santilli, Algebras, Groups and Geometries Vol. 10, 273-322 (1993).
and applied the new numbers in his monographs
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1993).
"Elements of Hadronic Mechanics"
Vol. II: "Theoretical Foundations"
R. M. Santilli, Ukraine Academy of Sciences (1994).
A readable presentation of Santilli's genonumbers in Italian is given by the paper
I nuovi numeri santilliani,
Parte II: I nuovi numeri genotopici ed iperstrutturali\\ e loro applicazioni
2.2C. Discovery of hypernumbers (1994)
Despite the above momentous discoveries, Santilli continued to remain dissatisfied because, as he stated in his works and correspondence, I cannot accept the idea that the DNA code can be understood with genonumbers because, even though they do represent the irreversibility of biological processes, they cannot possibly represent how two atoms of a DNA can produce an entire organ with a very large number of constituents.
In this way, the genonumbers were extended to yet new numbers today known as Santilli's hyperreal, hypercomplex and hyperquaternionic numbers to the right and to the left, or generically as hypernumbers that are multivalued, namely, not only the units and products to the right and to the left are different, but the hyperunit has an ordered set of values and, consequently, the multiplication yields an ordered set of results. For instance, the hyper-lifting of example (2.7) would yield expressions of the type
Santilli published his hypernumbers for the first time in the monograph
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
and then in the mathematical memoir
Nonlocal-integral isotopies of differential calculus,
mechanics and geometries
with applications to biology presented in the subsequent monograph
"Isotopic, Genotopic and Hyperstructural Methods in Theoretical Biology"
A recent mathematical presentation of hypernumbers, including its formulation via hyperstructural methods, can be found in Chapter 5 of the monograph
Hadronic Mathematics, Mechanics and Chemistry, Volume III:
Iso-, Geno-, Hyper-Formulations for Matter
2.2D. Discovery of isodual numbers (1993)
Despite all the above discoveries, each being quite significant, Santilli remained dissatisfied because, as he puts it in his works and correspondence: When I look at the stars, I feel very frustrated as a physicist for my complete inability to study whether a far-away star or quasar is made up of matter or of antimatter.
As indicated in Section 1.4, mathematical and physical methods of the 20th century were insufficient to allow any consistent classical description of antimatter. The new iso-, geno- and hyper-numbers were insufficient to reach the needed classical description of antimatter precisely because of their isomorphism to conventional numbers. In fact, charge conjugation is an anti-automorphism. Hence, a classical representation of antimatter admitting an operator image compatible with charge conjugation needs a mathematics that is anti-homomorphic or, better, anti-isomorphic to the conventional mathematics, as well as to its iso-, geno-, and hyper-liftings.
When at the Department of Mathematics of Harvard University, he conducted in the early 1980s a comprehensive search in the Cantabridgean mathematics libraries and concluded that the mathematics needed for a classical representation of antimatter did not exist in the form needed by physicists, such as to yield under quantization an image equivalent to charge conjugation. Consequently, the needed new mathematics had to be built.
A day in February 1982 Santilli invited one of his mathematics colleagues to visit the mathematics library (located in the ground floor of Harvard's Science Center) and suggested him to select any desired volume by opening it at any desired page. He would prove that the mathematics in that arbitrary volume in that arbitrary page would not allow a physically consistent classical representation of antimatter. He was indeed right.
As a physicist, Santilli was forced, again, to study yet new mathematics and, for that scope, he was forced to study yet new numbers. In this way, he discovered that the axioms of a numerical field admit negative units and the resulting fields are anti-isomorphic to conventional fields as desired. More generally he introduced a new map he called isoduality (denoted with an upper index d) consisting of an anti-Hermitean operation given for an arbitrary quantity Q(n, ...) by
As the simplest possible illustration, consider the conventional field F(n, x, I). Then, Santilli isodual field is given by
To illustrate the mathematical novelty, we can report the following episode quoted by Santilli in footnotes of some of his books. In June 1996, Santilli and his wife Carla went to Palermo, Sicily, to pay their tribute to the Circolo Matematico Palermo for the publication of a special issue of its famous mathematics journal entirely dedicated to Santilli's isotopies. During that occasion, as a gesture of appreciation, the Editor in Chief of the journal, Prof. P. Vetro, found a 20 minutes opening at a mathematics conference going on in Palermo at that time and suggested Santilli to present there his new mathematics just appeared in the Rendiconti.
Santilli accepted the offer and elected to present his recently discovered isodual number theory and related mathematics by initiated his lecture with the projection in the big screen of a transparency with only the number "-1" in it and the indication that he assumed that quantity as the basic unit of his mathematics. At that view and statement, the audience went into great agitation with numerous questions from all sides, often repeated various times, to such a disarray that 20 minutes passed without Santilli being able to present any additional transparency.
Mathematicians are accustomed to write structures in an abstract, realization-free form. For this purpose Santilli suggests the use of the conventional symbol F(n, x, I) for the abstract unification of all his new numbers, provided one has a knowledge of all possible realizations, not only of the unit, but also of the related multiplications.
The above abstract unification would cause serious problems if used in physics because, e.g., it could cause the inadvertent mixing of particles and antiparticles. This is the reason that in physics it is much better to have different specific formulations for fields, isofields, genofields, hyperfields and their isoduals, since the identification of the assumed numbers and their unit identifies the level of treatment and related applications.
We cannot close this section without an indication of yet another mathematical discovery by Santilli given by iso-, geno-, hyper-fields and their isoduals when the related generalized unit singular (or divergent), namely, are admitted to have a functional. dependence with null (or infinite) value, an occurrence simply impossible for the 20th century mathematics, since fields are assumed to have the trivial unit + 1. As we shall see in Chapter 3, this case is of particular physical relevance since Lim I* → 0 represents gravitational singularities.
To the Foundation's best knowledge, Santilli published for the first time his isodual numbers in the historical mathematics papers and monograph of 1993
Isonumbers and genonumbers of dimension 1, 2, 4, 8, their isoduals and "hidden numbers" of dimension 3, 5, 6, 7.
"Isodual Theory of Antimatter, with Application to Antigravity, Grand Unification and Cosmology",
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
A readable presentation of Santilli's isodual numbers in Italian is given by the article
I nuovi numeri santilliani,
Parte III: I nuovi numeri isoduali e loro applicazioni
2.3. DISCOVERY OF ISO-, GENO-, HYPER-DIFFERENTIAL CALCULI, FUNCTIONAL ANALYSIS AND THEIR ISODUALS (1996)
Santilli's main scientific objective has been the study of Lagrange's and Hamilton's legacy (Section 1.1), namely, the study of contact non-Hamiltonian interactions at all possible levels, from Newtonian mechanics to second quantization. Besides the need for new numbers, Santilli faced another major technical obstacle, that of achieving the representation of all possible (well behaved) non-Hamiltonian forces via an action principle, because such a principle is necessary for quantization. As a matter of fact, the lack of achievement of any quantum formulation of non-Hamiltonian interactions during the 20th century was precisely due to the lack of any action principle for their classical representation, with consequential lack of any consistent method for their quantization.
Again, as a theoretical physicist, Santilli was forced to study pure mathematics as a condition to formulate consistent physical theories. After decades of trials and errors, Santilli recalled in the mid 1990s that Newton had to invent (with Leibnitz) the differential calculus before he was in a position to write his celebrated equations.
In this way, Santilli inspected the differential calculus and discovered that, contrary to a deeply rooted belief in pure mathematics for over about centuries, the differential calculus is indeed dependent on the assumed basic unit. Let r be the coordinate of a Newtonian particle and dr its differential. Assume the isotopic lifting of r into an isocoordinate r* = r x I*, with isounit I* = 1 / T > 0. In this case, Santilli proved that the isodifferential and isoderivative are given by
The above studies marked the discovery of a structural generalization of the differential calculus that, as illustrated by the momentous implications outlined in this presentation, is indeed yet another mathematical discovery of clear historical proportions, today known as Santilli's so-, geno-, hyper-differential calculi for matter and their isoduals for antimatter.
It should be noted that the conventional differential calculus has only one formulation, the conventional one. By contrast, Santilli's generalized differential calculi have two formulations expressed in Eqs. (2.11)-(2.13) for the isotopic case, namely, a first formulation on isospaces over isofields, and a second one given by its projection on conventional spaces over conventional fields. Note that at the abstract representation-free level, isodifferential and conventional calculi coincide, and the same holds for the other calculi.
As we shall see in Section 3.12, Santilli's generalized differential calculi did permit the representation, for the first time, of all (well behaved) non-Hamiltonian Newtonian systems via a generalized action principle, thus permitting the identification, also for the first time, of their rigorous map into operator forms constituting the foundations of hadronic mechanics.
These various new calculi were first published in the 1995 second edition of the monograph
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
and in all subsequent works
Nonlocal-integral isotopies of differential calculus,
mechanics and geometries
The functional isoanalysis was initiated by Santilli with the isotopies of basic functions such as exponential, logarithm, trigonometric and other functions, see Chapter 6 of the monograph
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
The construction of the new isoanalysis was continued by the physicist J. V. Kadeisvili, e.g. in the papers
Elements of Functional Analysis
Elements of Fourier-Santilli isotransforms
Additional work was done by the physicists A. K. Aringazin et al, see Appendix 4.B of the monograph
"Elements of Hadronic Mechanics"
Vol. II: "Theoretical Foundations"
2.4. DISCOVERY OF ISO-, GENO-, HYPER-, SPACES AND THEIR ISODUALS (1983).
2.4A. Santilli's generalized spaces
The implications of these broader spaces are far reaching, as we shall see. Consider the conventional, (3+1)-dimensional Minkowski space M(r, m, I) with spacetime coordinates r = (rk), k = 1, 2, 3, 4, r4 = t, metric m = Diag. (1, 1, 1, - c2) and invariant r2 = (ri x mij x rj) x I where I is the unit of the Lorentz symmetry SO(3.1), I = Diag. (1, 1, 1, 1). Then, the isounit, isometric, and isoline element on Minkowski-Santilli isospace are given by
Michele Sacerdoti, to appear.
It should be indicated that Santilli's hypernumbers are different than those belonging to hyperstructures because the former use conventional operations while the latter use abstract operations. Also, Santilli's hypernumbers verify all axioms of a field, while conventional hyperstructures do not generally admit any unit at all, thus not being generally formulated over a field, with consequential severe restrictions in applications.
R. M. Santilli, Ukraine Academy of Sciences, 2nd edition (1995).
R. M. Santilli, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 7-82 (1996).
R. M. Santilli, Ukraine Academy of Sciences (1997).
R. M. Santilli, International Academic Press (2008)
provided that the above map is applied to the totality of the elements of a given theory and all its operations. This gave rise to: Santilli's isodual conventional, iso-, geno- and hyper-numbers; negative definite units called isodual conventional, iso-, geno-, and hyper-units; and corresponding multiplications called isodual conventional, iso-, geno-, and hyper-multiplications.
The isoduals of iso-, geno- and hyper-numbers can be similarly constructed via isoduality (2.9).
Even though seemingly trivial, isodual numbers have their own rather deep implications requiring attention to prevent inconsistencies. For instance, the statement of having +1,000 dollars in the bank, in reality means for isodual numbers that the account is 1,000 dollars in the red because the number +1,000 is now referred to the basic unit -1, the isodual norm of -1,000 is negative, etc.
R. M. Santilli, Algebras, Groups and Geometries Vol. 10, 273-322 (1993).
R. M. Santilli,Springer (2006).
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1993).
Michele Sacerdoti, to appear.
If the isounit is independent from the local variable of the calculus, the differential is indeed independent from the local valuable because
thus recovering the indicated belief in pure mathematics. However, when the isounit depends on local variable, I* = I*(r, ...), the above simplification is no longer possible because we have for the differential
with a corresponding complex relation for the isoderivative. The geno- hyper- and isodual versions are evidently characterized by the use of the corresponding generalized units. Note that the geno-, and hyper-differential calculi for matter and their isoduals for antimatter are particularly important for the correct treatment of irreversible processes, as we shall see in Chapters 3,4,5.
R. M. Santilli, Ukraine Academy of Sciences, 2nd edition (1995).
R. M. Santilli, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 7-82 (1996).
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1993).
J. V. Kadeisvili,
Algebras, Groups and Geometries Vol. 9, pages 283-318 (1992)
J. V. Kadeisvili,
Algebras, Groups and Geometries Vol. 9, pages 310-347 (1992)
R. M. Santilli, Ukraine Academy of Sciences (1994).
As it is well known, all quantitative studies are defined on a representation space, such as the Euclidean Minkowski Riemannian, Finslerian or other (metric or pseudo-metric) space that, in turn, is defined over a field of numbers. It is evident that the generalization of ordinary numbers produced a corresponding lifting of conventional spaces, today's known as Santilli's iso-, geno, and hyper- Euclidean, Minkowskian, Riemannian, Finslerian and other spaces for matter and their isoduals for antimatter.
where: we have shown the most general possible, diagonal realization of Santilli's isounit and its realization in physics via the so-called characteristic quantities nk, k = 1, 2, 3, 4; r4 = t x c; and the notation rk2, k = 1, 2, 3, 4, denotes the square of rk.
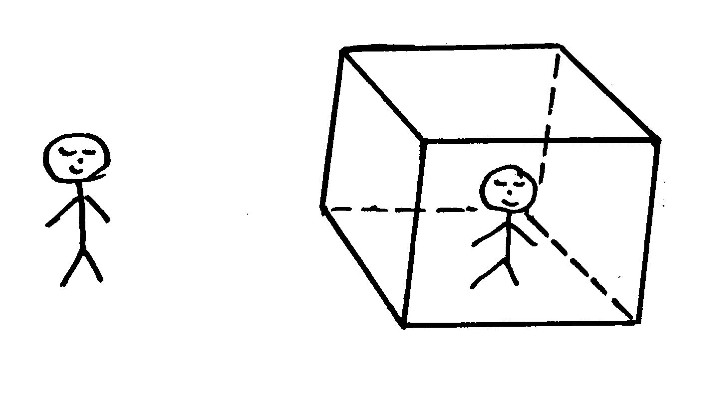
Figure 2.1: Santilli illustrates the far reaching implications of his isogeometries via the "isobox" consisting of a box inspected by two observers: an observer in the exterior verifying the conventional Minkowski spacetime, and an observer in the interior verifying the Minkowski-Santilli isospacetime. Santilli isonumbers and isospaces can be claimed to be understood if one understands that: the interior observer can be in the infinite future or past time with respect to the exterior observer; if the exterior observer sees a cube with 2 m side, the interior observer can see a room of dimension arbitrarily bigger or smaller compared to the exterior view; and if the exterior observer see a cube, the interior observer can see a cathedral. For explanations, interested readers should study the quoted literature.
2.4B. Preliminary implications
The implications of the above discovery can only be qualified as historical, as shown in the rest of this presentation. We only mention the achievement, for the first time in scientific history via a metric, of:
1) The representation of arbitrary speed of light C = c /n4, where n4 is the familiar index of refraction, with values C smaller (bigger) than c for physical media of low (high) density;
2) The representation of the actual dimension and shape of particles via the space-component n2k, k = 1, 2, 3 (normalized to the value 1 for the vacuum);
3) The representation of the density of the particles (or medium) considered via n42 (also normalized to the value 1 for the vacuum);
4) The representation of the inhomogeneity of the physical medium considered via, e.g., a dependence of the characteristic quantities on the distance and other variables, nk = nk(r, ...);
5) The representation of the anisotropy of physical medium considered via a different value of the characteristic quantities.
A fundamental property of Santilli isospaces is that, by conception and construction, they are isomorphic to the original spaces for all positive-definite isounits. In fact, at the abstract, realization-free level, there is no difference between the conventional Minkowski space and the Minkowski-Santilli isospace, to such an extent that they can be expressed via the same symbols, only subjected to different interpretations. As we shall see in the subsequent chapters, this feature has very important implications for numerous aspects of scientific knowledge.
Another important property is that Santilli's isospaces unify all possible spaces with the same dimension. In fact, isoline element (2.16) clearly includes as particular cases the Minkowskian, Riemannian, Finslerian, non-Desarguesian and other line elements. Hence, M*(r*, m*, I*) unifies all possible spacetimes in (3.1)-dimensions. In the event the positive-definiteness of the isounit is relaxed, M*(r*, m*, I*) unifies all possible 4-dimensional spaces, including the Euclidean one, the differentiation between one space and the other being set by the unit.
As we shall see, the above unification alone has far reaching implications, such as the achievement of the first and only known, axiomatically consistent grand unification of electroweak and gravitational interactions that had escaped the best minds of the 20th century, including Albert Einstein.
Santilli's geno- and hyper spaces have implications perhaps more intriguing than those of the isospaces, because the former provide the first known geometric representation of irreversibility by embedding the direction of time in the geno- and hyper-metric itself, while the new spaces remain isomorphic to the original space even though, quite remarkably, the geno- and hyper-metrics are not necessarily symmetric.
Isospaces were first presented in two historical papers of 1983 on the structural generalization of the Minkowski space, the Lorentz symmetry and special relativity, with classical representation in the paper
Lie-isotopic lifting of special relativity
for extended deformable particles
R. M. Santilli, Lettere Nuovo Cimento Vol. 37, 545-555 (1983),
and operator counterpart
Lie-isotopic lifting of unitary symmetries and
of Wigner's theorem for extended deformable particles
R. M. Santilli, Lettere Nuovo Cimento Vol. 38, 509-521 (1983).
A more detailed mathematical treatment via the isotopies of the Euclidean space was presented in the two papers of 1985 quoted earlier
Lie-isotopic liftings of Lie symmetries, I: General considerations
R. M. Santilli,
Hadronic J. Vol. 8, 25-35 (1985)
Lie-isotopic liftings of Lie symmetries, II: Lifting of rotations
R. M. santilli,
Hadronic J. Vol. 8, 36-51 (1985)
In reality Santilli wrote first the latter two papers on the iso-Euclidean space and then wrote the paper on the isotopies of Minkowski space. Unfortunately the former ended up being published two years following the publication of the latter due to incredible editorial obstructions Santilli felt obliged to report in the first 1985 paper.
Iso-, geno- and hyper-spaces for matter and their isoduals for antimatter were systematically presented in the historical memoir published by the Rendiconti in 1996, with the initiation of their topology
Nonlocal-integral isotopies of differential calculus,
mechanics and geometries
R. M. Santilli, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 7-82 (1996).
Comprehensive studies were then published in Santilli's various books, including
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences (1993),
"Elements of Hadronic Mechanics"
Vol. II: "Theoretical Foundations"
R. M. Santilli, Ukraine Academy of Sciences (1994)
Systematic mathematical studies on the new spaces and the the resulting new topology were conducted in the following monograph among various others studies (see the general bibliography)
Fundamentos de la Isoteoria de Lie-Santilli
Raul M. Falcon Ganfornina and Juan Nunez Valdes International Academic Press (2001)
2.4C. Iso-, geno-, hyper-topologies and their isoduals
The central mathematical tools of 20th century quantitative sciences have been the conventional differential calculus and topology which tools, being strictly local-differential, are the ultimate reason for the abstraction of particles as dimensionless points moving in empty space (conditions characterizing the {\it exterior dynamical problem in vacuum})\index{Exterior dynamical problem}
and related dramatic limitations, such as those originating from Theorem 1.1.
Since the initiation of his research in the late 1960s, Santilli's primary objective has been the study of extended, nonspherical and deformable particles in conditions of partial or complete mutual penetration (Figures 1.3, 1.4) or for extended particles moving within a physical medium (conditions characterizing the {\it interior dynamical problem within physical media})\index{Interior dynamical problem}.
It is evident that such a study could not be conducted in a consistent way without the prior lifting of the local-differential character of the 20th century mathematics into a suitable nonlocal-integral form. This problem occupied Santilli's mind for decades due to the lack of the new mathematics in the form needed for physical applications, that is, as a covering of conventional local-differential methods. Said covering character was needed to recover 20th century physics when particles exit physical media and return to move in vacuum. Following extensive search in Italian and American mathematics libraries, Santilli was unable to locate the indicated new mathematics in the needed covering form. Therefore, the new mathematics had to be built prior to any quantitative physical, chemical or bio;logical study.
After decades of attempts, Santilli finally succeeded to lift the local-differential calculus in the covering nonlocal-integrodifferential form (Section 2.3) as needed for physical applications, by embedding all nonlocal-integral terms in the isounit $\hat I(t, r, p, ...) > 0$ as in Eq. (2.11), so as to recover the conventional differential calculus as a trivial particular cases when $\hat I = I$ [loc. cit.].
Being primarily interested in physical applications, Santilli contacted various mathematicians and suggested them to study the isotopy of the conventional topology, such a the isotopy the conventional {\it Euclidean topology}\index{Euclidean topology} at the foundation of both the Galilean and special relativities and quantum mechanics. The mathematicians Gr. T. Tsagas (then Chairman of the Department of Mathematics of Aristotle University, Thessaloniki, Greece) and D. S. Sourlas (also from Greece) answered Santilli's call and initiated the construction of the proposed {\it isotopology}\index{Isotopology} in the early 1990s
"Mathematical Foundation of the Lie-Santilli Theory"
D. S. Sourlas and G. T. Tsagas,
Ukraine Academy of Sciences 91993)
However, these initial versions were formulated on a conventional field, thus preventing consistent applications to physics (due to the Theorems of Catastrophic Inconsistencies of Section 3.7). Consequently, Santilli addressed the problem in 1996
Nonlocal-integral isotopies of differential calculus,
mechanics and geometries
R. M. Santilli, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 7-82 (1996).
and achieved the first known formulation of the isotopology on an isospace over an isofield for the characterization of matter interior problem, as well as its isodual for antimatter interior problem. In memoir [loc. cit.], Santilli also proved the intrinsic capability of the isotopology to characterize extended, nonspherical and deformable particles via realizations of the isounit of type (2.14), thus completing the construction of the new mathematics needed for basic physical, chemical and biological advances.
Subsequently, comprehensive studies on the isotopology were conducted in early 2000s by the mathematicians R. M. Falcon Ganfornina and J. Nunez Valdes of the Department of Mathematics of the University of Seville, Spain, who achieved the final form of the nonlocal-integrodifferential topology used by Santilli in all applications for matter, and today known as the Tsagas-Sourlas-Santilli-Ganfornina-Valdes isotopology or TSSGN isotopology for short.\index{Isotopology.
The best and most comprehensive mathematical reference on the TSSGN isotopology, including a review and quotation of all preceding literature, is the monograph of 2001 by R. M. Falcon Ganfornina and J. Nunez Valdes
Fundamentos de la Isoteoria de Lie-Santilli
Raul M. Falcon Ganfornina and Juan Nunez Valdes International Academic Press (2001)
that essentially reports the Ph. D. Thesis of the first author under the guidance of the second author. Ironically, during the defense of the Ph. D. Thesis, both the student and his teacher were attacked for apparent triviality of the results, because the covering isotopology can be expressed by putting a "hat" on all symbols of the conventional topology.
However, as indicated in the Foreword of this chapter, besides momentous mathematical advances, the new isotopology allows the extension of Einstein's axioms, from their point-like abstraction of particles moving in vacuum, to extended particles in conditions of total or partial mutual penetration, as occurring in the structure of hadrons, nuclei and stars, with consequential prediction and quantitative treatment of much needed new clean energies that are inconceivable with the old topology (Chapter 9).
for which reason we included comments in the Foreword of this chapter.
The best known presentation of the isodual TSSGN isotopology for antimatter is that in Santilli's monograph
"Isodual Theory of Antimatter, with Applications to Antigravity, Grand Unification and Cosmology,"
R. M. Santilli,
Springer (2006)
A recent readable presentation of these new topologies for physicists is that available in Volumes II and III of the 2008 monographs
Hadronic Mathematics, Mechanics and Chemistry, Volumes I, II, III, IV and V:
R. M. Santilli, International Academic Press (2008)
At this writing (Springs 2009), only the TSSGN isotopology for matter and its isodual for antimatter are known, while their geno- and hyperformulations and related isoduals are unknown, although expected to be a natural extension of the indicated isotopology and its isodual. Their study by interested mathematicians is solicited and financially supported by the Santilli Foundation due to their dramatic implications, such as setting mathematical foundations for the first known rigorous connection between irreversible geno-mechanics and thermodynamics.
2.5. DISCOVERY OF ISO-, GENO-, HYPER-SYMPLECTIC GEOMETRIES AND THEIR ISODUALS (1996)
As it is well known, the symplectic geometry allows one of the most rigorous studies of classical Hamiltonian systems, as well as their quantization. Hence, Santilli could not escape a re-inspection of the symplectic geometry because his main physical objective was to represent the most general possible (sufficiently smooth) non-Hamiltonian systems.
Consider the conventional canonical symplectic structure on a cotangent bundle with local charts r and p on the reals, ω = dp ∧ dr. It was easy for Santilli to formulate its isotopic covering on an isocotangent bundle by first showing that local isochart is given by r* = rxI*, p* = pxI*-1 on the isoreals, with consequential isocanonical two isoform
that, as one can see, coincides with the conventional canonical two-form for all constant isounits, but possesses otherwise dramatic differences with the conventional version because it does allow the desired representation of all well-behaved equations of motion with all possible potential and nonpotential forces.
These studies lead to what are today known as Santilli's iso-, geno-, and hyper-symplectic geometries for matter and their isoduals for antimatter. Their most salient feature is that of coinciding with the conventional symplectic geometry at the abstract level to such an extent that Santilli insists in writing the equations for his covering geometries via the symbols of the conventional geometry, and merely subjects them to a broader interpretation.
The above coverings of the symplectic geometry were first published in the monograph
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences (1993),
as well as in his mathematical memoir
Nonlocal-integral isotopies of differential calculus,
mechanics and geometries
R. M. Santilli, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 7-82 (1996).
2.6. ISOTOPIC UNIFICATION OF MINKOWSKIAN, RIEMANNIAN AND FINSLERIAN GEOMETRIES (1998)
A very special feature of Santilli's isotopies is that of unifying seemingly different structures into a covering form that enjoys the basic property of invariance. Following the achievement in 1983 of his iso-Minkowski spaces (Section 2.4), Santilli realized that there is no difference between his iso-Minkowski metric m*(r, ...) and a conventional Riemannian metric g(r) or a Finslerian metric g(r, v, ...)since the explicit form of the characteristic quantities ni is unrestricted by the isotopies (only their positive-definite character is requested for isotopies).
But Santilli knew at that time (early 1990s) that the iso-Minkowski spaces are isomorphic to the conventional space. Hence, his isotopic methods offered a unique possibility of unifying the Minkowskian and the Riemannian geometries, with far reaching implications, such as the first axiomatically consistent grand unification of electroweak and gravitational interactions reviewed in the physics chapters.
In this way, Santilli achieved a new geometry on isospaces over isofields, today called Minkowski-Santilli isogeometry equipped with all the machinery of the Riemannian geometry (such as covariant derivative, Christoffel's symbols, etc.), that does unify the Minkowskian and Riemannian geometries, while admitting both as particular cases depending on the selected isounit.
This additional historical achievement was published by Santilli in various works, with primary presentation in the memoir
Isominkowskian geometry for the gravitational treatment of matter
and its isodual for antimatter
R. M. Santilli, Intern. J. Modern Phys. D Vol. 7, 351-407 (1998).
Numerous papers then appeared showing the so called "direct universality" of the Minkowski-Santilli isogeometry, that is, the capability of admitting as particular cases all infinitely possible (non singular) geometries on a (3+1)-dimensional space (universality), directly in the selected coordinates without any need for the transformation theory (direct universality). Among numerous papers on this aspect, we quote the following
Universality of Santilli's iso-Minkowskian geometry
A. K. Aringazin and K. M. Aringazin, in "Frontiers of Fundamental Physics"
M. Barone and F. Selleri, Editors, Plenum 91995)
2.7. LIE-ISOTOPIC COVERING OF LIE'S THEORY AND ITS ISODUAL (1978)
As it is well known, Lie's theory has been the fundamental mathematical tool of the 20th century quantitative sciences, thus having been the subject of vast attention and having achieved a vast diversifications into various branches, such as:
1) The universal enveloping associative algebra U over a field F(n, x, I) as a vector space whose elements are: the unit matrix I = Diag. (1, 1, ..., 1) with the dimension of the selected representation; the N (Hermitean) generators Gk, k = 1, 2, 3, ..., N, with conventional associative products Gi x Gj; and the infinite-dimensional basis characterized by the Poincare'-Birkhoff-Witt theorem via the ordered monomials
which basis is necessary for the definition of exponentiation W = exp (G x w x i), where w ε F, and other operations on U;
2) The N-dimensional Lie algebra L which is the antisymmetric algebra U- attached to U with Lie product and closure relations
where the Cs are the structure constants of L;
3) The Lie group g whose realization most important in physics is that of Lie's transformation groups of a quantity Q(w) that can be written in the following finite and related infinitesimal forms
plus the representation theory generally constructed either on a right acting module u or, equivalently for Lie's theory, the left acting module -u,
It should be recalled that Lie's theory characterizes the fundamental dynamical equations of quantum mechanics, those of the time evolution via Eqs. (2.21) with w representing time t. Lie's theory also characterizes all fundamental symmetries in physics, such as the Lorentz and Poincare' symmetries at the foundation of special relativity, the SU(3) symmetry for the classification of particles, etc.
Immediately following the study of Lie's theory during his graduate studies in physics in Torino, Italy, in the late 1960s, Santilli realized the excessive limitations of the theory, since Lie's theory solely applies for systems that are linear, local-differential and Hamiltonian (canonical at the classical level and unitary at the operator level), while the systems of the real world are generally nonlinear, nonlocal-integral and admit both Hamiltonian and non-Hamiltonian interactions. Hence, Santilli set in the late 1960s his goal to reach a structural generalization of Lie's theory applicable to a broader class of systems.
As a main result of his Ph. D. thesis, Santilli published in 1967 the first formulation in physics records of the Lie-admissible covering of Lie's theory studied in the next section that is ideally suited for rigorous formulations of irreversibility as we shall see in Chapter 3.
However, Santilli knew of the existence in nature of systems that are non-Hamiltonian, yet verify all conventional conservation laws (see Section 3.12), whose characterization requires an algebra which is a covering of Lie algebras (to exit from the class of Hamiltonian equivalence), yet it is characterized by an antisymmetric product (to characterize the conservation of total quantities). Lie-admissible algebras do not verify this requirement because, as we shall see in the next section, their product is neither totally antisymmetric nor totally symmetric, thus being particularly suited to represent time rate of variations of physical quantities, but not their conservation.
Following decades of silent research, Santilli released in 1978 a particular case of the broader Lie-admissible theory consisting of an isotopic (axiom-preserving) generalization (he called lifting) of all branches of Lie's theory, today known as the Lie-Santilli isotheory,, that constitutes one of the biggest mathematical and physical discoveries of the 20th century, not only because of fundamental mathematical novelty, but also because of its predictable far reaching implications for all quantitative sciences, as shown in Chapters 3, 4, 5.
Santilli's Lie-isotopic theory is based on all preceding mathematical discoveries, that is, its correct formulation requires isofields, isospaces, isodifferential calculus, isofunctional analysis, etc., to such an extent that the lack of isotopic lifting of only one methodological aspect of Lie's theory causes catastrophic inconsistencies (lack of invariance of the theory under its own action, etc.). In fact, the mixing of Lie and Lie-Santilli's methods would be like formulating the conventional Lie's theory on Santilli's isofields, resulting in evident inconsistencies.
Under the above understanding, the presentation of Santilli's Lie-isotopic theory is now (following its discovery) rather elementary and its main branches can be summarily presented for applied mathematicians as follows:
1*) The universal enveloping isoassociative algebra U* over an isofield F*(n*, x*, I*) as a vector space whose element are: the isounit I* = 1/T > 0 (where the positive-definiteness is assumed to preserve Lie's axioms and the dimension is that of the used isorepresentation); the same (Hermitean) generators Gk, k = 1, 2, 3, ..., N, of Lie's theory with isoassociative product and related isounit
and the infinite dimensional isobasis characterized by the Poincare'-Birkhoff-Witt-Santilli isotheorem with ordered isomonomials
permitting the definition of isoexponentiation
and other operations on U*;
2*) The N-dimensional Lie-Santilli isoalgebra L* which is the antisymmetric isoalgebra U*- attached to U* with Lie-Santilli isoproduct and closure relations
where Cij*k characterizes the isostructure isofunctions of LCijk with constant particularizations;
3*) The Lie-Santilli isogroups g* whose realization most important in physics is that of Santilli's isotransformation isogroups of a generic quantity Q*(w*) on U* over F* that can be written in the following finite and infinitesimal forms each in a dual way, the formulation on U* over F* and its projection on U over F
plus the isorepresentation isotheory generally constructed either on a right acting isomodule u* or, equivalently, the left acting isomodule -u*.
As one can see, Santilli's isotheory causes the emergence of a generally nonlinear, nonlocal and non-Hamiltonian operator T in the exponent of the isotransformations, as well as in the broader isobrackets of the infinitesimal transforms, thus permitting indeed the originally desired extension of Lie's theory to nonlinear, nonlocal and non-Hamiltonian systems with far reaching implications indicated in the subsequent chapters.
Remarkably, Santilli proved the "direct universality" of his isotheory for all well behaved nonlinear, nonlocal and non-Hamiltonian systems via the following:
THEOREM 2.7.1: All sufficiently smooth nonlinear, nonlocal-integral and non-Hamiltonian systems (whether classical noncanonical or operator nonunitary) on conventional spaces over a conventional field always admit an isounit for which they can be identically reformulated on isospaces over isofields where they are isolinear, isolocal and isocanonical or isounitary (verify the axioms of linearity, locality and Hamiltonian character on isospaces over isofields).
The reconstruction of linearity, locality and canonicity or unitarity is merely done by embedding all nonlinear, nonlocal and non-Hamiltonian terms in the isounit. This important property is the conceptual essence of Santilli's isotheory in both its mathematical meaning and physical applications. Recall that Lie's theory is strictly linear, local-differential and Hamiltonian on conventional spaces over ordinary fields. In the event Santilli had not preserved at the covering level these fundamental properties, his theory could not be called an "isotopy" (axiom-preserving) lifting of Lie's theory. In turn, the loss of the isotopic character would have caused serious physical problems.
Recall from the preceding sections that the isotopies have the important capabilities of unifying seemingly different mathematical structures. In the original proposal of 1978, Santilli proved that the Lie-isotopic algebra O*(3) unifies all simple (compact and non-compact) Lie-algebra of dimensions three, and then formulated the following:
CONJECTURE 2.7.1: All simple Lie-algebras of dimension N can be unified into one single simple Lie-isotopic algebra of the same dimension.
The mathematician Gr. Tsagas proved the above conjecture to be correct for all simple Lie algebras with the exclusion of the exceptional algebras (see the reference below). The Foundation is interested in funding the completion of the proof of Conjecture 2.7.1 by qualified mathematicians.
The axiomatic unity of the conventional Lie theory and its isotopic covering is such that Santilli insists in presenting the latter with the same symbols of the former, only subjected to a broader realization, as it is the case for isonumbers, isospaces, isogeometries, etc.
As we shall see, the above property also differentiates Santilli's studies from a variety of other attempts to generalize Lie's theory, all known today to verify the Theorems of Catastrophic Mathematical and Physical Inconsistencies recalled in Section 3.9, precisely because of the latter theories are based on the broadening of Lie's theory, on one side, combined with the preservation of the conventional mathematics, on the other side.
The above isotopic lifting of Lie's theory was constructed by Santilli for the sole treatment of matter. For the classical treatment of antimatter in such a way to achieve compatibility with the operator formulations, Santilli needed an anti-isomorphic image of the above Lie-isotopic theory that he constructed via his isodual map (2.9) applied to the totality of quantities and their operations of the Lie-isotopic theory. This resulted in two new coverings of Lie's theory today known as Santilli isodual Lie theory and isodual Lie-isotopic theory, that are not reviewed here for brevity.
The discovery of the isotopic covering of each branch of Lie's theory was published for the first time in 1978 when Santilli was at Harvard University under DOE support via the two hundred pages historical memoir
On a possible Lie-admissible covering of Galilei's relativity
in Newtonian mechanics for nonconservative and Galilei form-noninvariant systems
R. M. Santilli, Hadronic J. Vol. 1, 223-423 (1978).
The theory was then expanded in the series of volumes published by the most prestigious scientific house of the time, Springer Verlag, in its most prestigious series of Text and Monograph in Theoretical Physics,
"Foundations of Theoretical Mechanics, I: The Inverse Problem in Newtonian Mechanics"
R. M. Santilli, Springer-Verlag (1978)
"Foundations of Theoretical Mechanics, II: Birkhoffian Generalization of hamiltonian Mechanics"
R. M. Santilli, Springer-Verlag (1982).
These original presentations were based on isospaces, but defined on conventional fields. Subsequently, Santilli discovered the lack of completion of this formulation and, following the availability of the isonumbers, reached a mathematically consistent formulation in various works, such as in the monograph that included a treatment of isodual Lie theory and isodual Lie-isotopic theory
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1993),
"Elements of Hadronic Mechanics"
Vol. II: "Theoretical Foundations"
R. M. Santilli, Ukraine Academy of Sciences. 1st edition (1993)
The discovery of the isodifferential calculus permitted Santilli to achieve the final formulation of his Lie-isotopic theory that was published in the second edition of 1995 of the above two volumes. A comprehensive presentation of the isodual Lie theory and the isodual Lie-isotopic theory is available in the monograph
"Isodual Theory of Antimatter, with Application to Antigravity, Grand Unification and Cosmology",
R. M. Santilli, Springer (2006)
Santilli's most recent presentation is available in the monograph with a treatment of the Lie, Lie-isotopic theories and their isoduals
Hadronic Mathematics, Mechanics and Chemistry, III:
Iso-, Geno-, Hyper-Formulations for Matter
and Their Isoduals for Antimatter
R. M. Santilli, International Academic Press (2008)
Due to its historical importance, the Lie-Santilli isotheory has been the subject of numerous independent studies, among which we can quote the review papers
An introduction to the Lie-Santilli isotopic theory
J. V. Kadeisvili, Mathematical Methods in Applied Sciences Vol. 19, 1349-1395 (1996)
Foundations of the Lie-Santilli isotheory
J. V. Kadeisvili, Rendiconti Circolo Matematico Palermo, Suppl. Vol. 42, 83-135 (1996).
and the monographs
"Mathematical Foundation of the Lie-Santilli Theory"
D. S. Sourlas and G. T. Tsagas, Ukraine Academy of Sciences 91993).
"Santilli's Isotopies of Contemporary Algebras
Geometries and Relativities",br>
J. V. Kadeisvili, Ukraine Academy of Sciences, Second edition (1997)
Fundamentos de la Isoteoria de Lie-Santilli
Raul M. Falcon Ganfornina and Juan Nunez Valdes International Academic Press (2001)
The proof of Conjecture 2.7.1 for all simple Lie algebra with the exclusion of the exceptional algebras can be found in the paper
Studies on the classification of Lie-Santilli algebras,
G. Tsagas, Algebras, Groups and Geometries Vol. 13, pages 129--148 (1996).
For a comprehensive list of all contributions on the Lie-Santilli isotheory, the interested scholar is suggested to consult the
General Bibliography on Santilli Discoveries
2.8. LIE-ADMISSIBLE COVERING OF THE LIE-ISOTOPIC THEORY AND ITS ISODUAL (1967)
Remarkably, Santilli remained dissatisfied with his own Lie-isotopic theory for physical and not mathematical reasons. Due to its structure and underlying topology, Lie's theory is ideally suited to represent a closed-isolated system of particles that, being necessarily abstracted as point-like, have no collisions, thus characterizing a Hamiltonian system (namely, a system entirely described by the Hamiltonian). This is typically the case for the atomic structure and other systems. In these cases, the antisymmetric character of Lie's brackets [A, B] = A x B - B x A = - [B, A] permits the representation of the conservation of the total quantities (represented in physics by the generators), as it is the case for the Hamiltonian
Santilli's Lie-isotopic theory does enlarge the class of represented systems into particles that are extended (see Section 3.12), thus experiencing collisions with both Hamiltonian and non-Hamiltonian interactions, yet the systems are isolated from the rest of the universe, thus also verifying total conservation laws. This is the case at a classical level of the structure of a planet such as Jupiter, or a nucleus at the operator level. Since Santilli's isotopic product is also antisymmetric, [A, B]* = A x T x B - B x T x A = - [B, A]*, it allows total conservation laws, such as
where H is the conventional Hamiltonian and T represent all contact non-Hamiltonian interactions and effects (see Chapter 3).
Hence, the Lie-isotopic theory cannot be a final theory because the systems of the physical reality are, in general, open, nonconservative and irreversible, as it is the case for a constituent of Jupiter or a proton in the core of a star when considering the rest of the system as external.
Santilli then searched for a covering of Lie's isotopic theory with a product (A, B) that is neither totally antisymmetry not totally symmetric, (A, B) ≠ ± (B, A) as a condition to characterize time-rate-of-variations f(t) of physical quantities,
since conservation laws are a trivial particular case.
While doing his Ph. D. studies at the University of Torino,. Italy, in the late 1960s, Santilli conducted for years a comprehensive search at European mathematical libraries to identify the desired covering of Lie's theory. He was finally rewarded with the identification of a paper of 1947 by the American mathematician A. A. Albert who introduced, without any specific realization or elaboration, the notion of Lie-admissible algebras as a (generally nonassociative) algebra U with abstract elements a, b, c, ... and abstract (generally nonassociative) product ab such that the attached algebra U- given by the same vector space as U but equipped with the product [a, b] = ab - ba, is Lie.
Albert also introduced the notion of Jordan-admissible algebra as the same algebras U when such that the attached algebra U+ with product {a, b} = ab + ba is Jordan. Following additional extensive library search, Santilli could only identify in European mathematics libraries a second note in the field by M. Tomber, although without realizations or elaborations.
Inspired by Albert's paper, Santilli published in 1967 the following paper on the embedding of Lie algebras in Lie-admissible algebras verifying central condition (2.31)
Embedding of Lie-algebras in nonassociative structures
R. M. Santilli, Nuovo Cimento Vol. 51, 570-576 (1967).
where he presented for the first time a specific realization of Lie-admissible and Jordan-admissible algebras with product (A, B) = p x A x B - q x B x A identified in more details in Section 3.8.
To understand the novelty of this paper (and others by Santilli written in 1967-1968 not quoted here for brevity), we recall that in 1967 Santilli moved from the University of Torino, Italy, to the University of Miami, Coral Gables, Florida, for a one year stay. During that time, he applied for a job to virtually all Departments of Physics in the U. S. A. by presenting with pride his discovery of Lie-admissible and Jordan-admissible algebras and their applications for the characterization of the time rate of variation of physical quantities. To his demise, no physicist in the U. S. A. knew the existence or meaning of these algebras at that time.
Numerous applications for a job at various U. S. Departments of Mathematics turned out also to be sterile because of the general lack of knowledge by mathematicians of the time of the algebras herein considered. In fact, the above 1967 paper was the very first on Lie-admissible and Jordan-admissible algebras in the physic literature and it was the mere third paper in the field in the mathematics literature, including the two preceding papers by Albert and Tomber duly quoted in the above listed reference.
In this way, Santilli understood that there was no possibility to secure an academic job in the USA with so advanced a research. He then turned his attention to orthodox lines of studies and soon got a position at Boston University. During the subsequent ten years, Santilli published excellent but fully aligned papers at Phys. Rev. MIT Annals of Physics and orthodox journals of that nature.
It was only in 1978 that Santilli decided to return to his "first scientific love" and released his studies on Lie-admissible algebras in the historical 200 pages long memoir quoted in Section 2.7. He subsequently developed further these studies resulting in a covering of his Lie-isotopic theory today known as Santilli's Lie-admissible theory (or genotheory), that is based on the preceding discoveries of genofields, genospaces, genodifferential calculus, etc. and can be outlined via the following branches:
b1f) An enveloping genoassociative algebra to the right (forward) Uf with ordered product to the right over the genofield to the right Ff(nf, xf, If) with elements given by: the genounit to the right If = 1/S; the generators Gk, k = 1, 2, ...,. N (as for the original Lie algebra), ordered genoassociative products to the right and related genounit
the infinite-dimensional genobasis acting on a genomodule to the right ur
and related genoexponentiation to the right
plus an enveloping genoassociative algebra to the left (backward) bU on the genofield to the left bF(bn, bx, bI) with elements: the genounit to the left bI = 1 / R; the generators kG (ordered to the left), k = 1, 2,..., N, with genoproduct and genounit
the infinite dimensional genobasis acting on a genomodule to the left bu (where now ub ≠ ± lu)
genoexponentiation to the left
and subsidiary condition
that are important in physical applications, e.g., to connect consistently motions forward and backward in time. The combination of the two genoenvelopes bU x Uf then acts on the genobimodule bu x uf which is the representation genospace of the theory.
b2f) Santilli's Lie-admissible algebras bLf as the bimodular algebra attached to bU x Uf characterized by the new product and closure rules
where the last expression is the projection of the algebra in the space of the original Lie algebra. As one can see, the resulting new product, here generically written (A, B) = A x R x B - B x S x A, is indeed jointly Lie-admissible and Jordan admissible although in Santilli's isotopic sense because
b3f) Santilli genotransformation groups bgf characterized by the left and right genoexponentiations (here written for simplicity in the representation space of the original Lie algebra)
,p>
with infinitesimal version characterized precisely by Santilli Lie-admissible brackets in the following simplified form
where G and w are the same generator and parameter as those of the attached Lie-isotopic algebra.
Unexpectedly, Santilli proved that his Lie-admissible covering of his Lie-isotopic theory is isomorphic to the Lie-isotopic and conventional Lie theory despite the lack of totally antisymmetric character of the product. This property was proved by noting that each of the two genoassociative algebras bU and Uf, when defined on the respective modules bu and uf over the corresponding genofields bF and Ff are isomorphic to the conventional associative enveloping algebra U over F.
The understanding of this important mathematical property, rather crucial for quantitative representations of irreversibility, can be seen by noting that, when the two products A x R x B and B x S x A are considered with respect to the conventional unit I of Lie's theory, the two algebras with products (A, B) = A x R x b - B x S x A and [A, B] = A x B - B x A are manifestly non-isomorphic.
However, when the product A x R x B is computed with respect to the genounit If = 1/R, the result is equivalent to that of the product B x S x A represented with respect to the genounit bI = 1/S, and both products are equivalent to the product A x B with respect to the unit I.
Santilli decomposed his Lie-admissible product into a totally antisymmetric and a totally symmetric forms,.
= (A x Y x B - B x Y A) + (A x Z x B + B x Z x A), R = Y + Z, S = Y - Z,
and proved the following important
THEOREM 2.8.1: Lie-admissible algebras with product (A, B) are "directly universal," in the sense of admitting as particular cases all possible algebras on a field of characteristic zero (universality) without use of the transformation theory (Direct universality).
In fact Santillis Lie-admissible algebras contain as particular cases all known or otherwise possible algebras with a bilinear composition law, such as: associative, flexible, alternative, Lie, Jordan, Lie-isotopic, Jordan-isotopic, supersymmetric and any other possible algebra.
The above presentation is solely intended for Lie-admissible treatment of matter in irreversible conditions. For the corresponding treatment of antimatter we have Santilli's isodual Lie-admissible theory, that can be constructed via the application of the isodual map (2.9) to the totality of the quantities and their operation of the Lie-admissible theory.
The Lie-admissible covering of the Lie-isotopic theory was presented in the historical 200 pages long memoir quoted above
On a possible Lie-admissible covering of Galilei's relativity
in Newtonian mechanics for nonconservative and Galilei form-noninvariant systems
R. M. Santilli, Hadronic J. Vol. 1, 223-423 (1978)
with physical applications presented in the joint memoir submitting hadronic mechanics as a covering of quantum mechanics
Need of subjecting to an experimental verification the validity within a hadron of Einstein special relativity and Pauli exclusion principle
R. M. Santilli, Hadronic J. Vol. 1, 574-901 (1978).
Santilli then developed further his Lie-admissible theory in the two volumes
"Lie-Admissible Approach to the Hadronic Structure, I: Non applicability of the Galilei and Einstein Relativities,"
R. M. Santilli, Hadronic Press (1978)
Lie-Admissible Approach to the Hadronic Structure, II: Coverings of the Galilei and Einstein Relativities"
R. M. Santilli, Hadronic Press (1982)
Additional presentations were made by Santilli in the two monographs published by Springer-Verlag in 1978 and 1982; a recent update is available in the 2008 monograph
"Foundations of Theoretical Mechanics, I: The Inverse Problem in Newtonian Mechanics"
R. M. Santilli, Springer-Verlag (1978)
"Foundations of Theoretical Mechanics, II: Birkhoffian Generalization of Hamiltonian Mechanics"
R. M. Santilli, Springer-Verlag (1982).
Subsequently, Santilli wrote a comprehensive presentation of his Lie-admissible theory and its isodual in the two monographs
"Elements of Hadronic Mechanics", Vol. I: "Mathematical Foundations"
R. M. Santilli, Ukraine Academy of Sciences. 1st edition (1993),
"Elements of Hadronic Mechanics"
Vol. II: "Theoretical Foundations"
R. M. Santilli, Ukraine Academy of Sciences, 1st edition (1994)
A more recent presentation is available in the monograph
Hadronic Mathematics, Mechanics and Chemistry, Vol. III: Iso-, Geno-, Hyper-Formulations for Matter
and Their Isoduals for Antimatter
R. M. Santilli, International Academic Press (2008),
and the following memoir published by the Italian Physical Society where Santilli applies his Lie-admissible theory for the first and only known invariant representation of irreversibility for matter and, separately, for antimatter, originating at the most elementary level of nature, that of elementary particles and antiparticles
Lie-admissible invariant representation of irreversibility for matter and antimatter at the classical and operator level
R. M. Santilli, Nuovo Cimento B Vol. 121, p. 443-595 (2006)
The most active mathematician in the study of Santilli's Lie-admissible algebras has been H. C. Myung,, e.g., with the monographs
"Lie Algebras and Flexible Lie-Admissible Algebras"
H. C. Myung,
Hadronic Press (1982)
"Malcev-Admissible Algebras"
H. C. Myung,
Birkhauser (1986)
"Mutations of Alternative Algebras"
A. Elduque and H. C. Myung,
Kluwer academic Publisher (1994)
Numerous mathematical works on lie-admissibility can be located in the
General Bibliography on Santilli Discoveries
2.9. INTEGRABILITY CONDITIONS FOR THE EXISTENCE OF A LAGRANGIAN
2.9A. Integrability conditions in Newtonian mechanics (1978).
Virtually the entire scientific production of the 20th century was based on the use of Lagrangian or Hamiltonian representations of Newtonian systems, then extended to operator formulations. Beginning with his graduate studies, Santilli set himself up to broaden these representations so as to avoid excessive abstractions and simplifications of reality, since said representations apply for point-like approximation of particles with sole action-at-a-distance interactions.
Hence, as part of his program, Santilli conducted a comprehensive study of the broadest possible systems representable via a Lagrangian or a Hamiltonian, and conducted this study via a systematic analysis of the integrability conditions for the existence of a Lagrangian or a Hamiltonian for given dynamical systems, called conditions of variational selfadjointness.
By indicating with the symbol SA (NSA) forces or equations verifying (violating) the conditions of variational selfadjointness, Santilli writes Newton's equations in a form decomposing forces into a component derivable from a potential (SA) and a second term representing all forces not derivable from a potential )(NSA)
and writes Hamilton's equations (1.2) in the corresponding form
As we shall see in Chapter 3, Santilli's mathematics allows the reformulation of Newton's equation into an identical selfadjoint form merely formulated on generalized spaces over generalized fields. The regaining of selfadjointness permits the recovering an action function with consequential means for a rigorous map to contact non-Hamiltonian interactions into operator forms, with endless applications.
These studies resulted in the two monographs indicated earlier publisher by Springer-Verlag, written when he was at Harvard University, with the most comprehensive references in the field up to 1982 that required Santilli one full year of search in the Cantabridgean libraries (an impeccable ethical conduct that is per se a great rarity in the contemporary widespread plagiarisms in science),
"Foundations of Theoretical Mechanics, I: The Inverse Problem in Newtonian Mechanics"
R. M. Santilli, Springer-Verlag (1978)
"Foundations of Theoretical Mechanics, II: Birkhoffian Generalization of Hamiltonian Mechanics"
R. M. Santilli, Springer-Verlag (1982).
2.9B. Integrability conditions in field theory (1975)
As indicated earlier, Santilli conceived first in 1967 his Lie-admissible theory and then studied its Lie-isotopic particularization. He did the same for the conditions of variational selfadjointness. In fact, when he was at the Center for Theoretical Physics of the Massachusetts Institute of Technology in the mid 1970s, Santilli first studied the integrability conditions for the existence of a Lagrangian or a Hamiltonian for the most general possible tensorial field equations, and published their simplification for Newtonian systems reviewed above, written subsequently when he was a Harvard University.
These studies produces the following three memoirs that constitute the most serious scholar works in the field ands remain grossly unsurpassed to this day in their essential results and mathematical rigor
Necessary and sufficient conditions for the existence of
a Lagrangian in field theory, I: Variational approach to
selfadjointness for tensorial field equations
R. M. Santilli, (MIT) Ann. Phys. Vol. 103, 354-408 (1977)
Necessary and sufficient conditions for the existence of
a Lagrangian in field theory, II: Direct analytic representation of tensorial field equations
R. M. Santilli, (MIT) Ann. Phys. Vol. 103, 409-468 (1977)
Necessary and sufficient conditions for the existence of
a Lagrangian in field theory, III: Generalized analytic representations of tensorial field equations
R. M. Santilli, (MIT) Ann. Phys. Vol. 105, 227-258 (1978)
**********************************